Comparing Fractions Worksheets⁚ A Comprehensive Guide
Comparing fractions is a fundamental skill in mathematics, and worksheets can be an effective tool for helping students master this concept. This comprehensive guide will explore the various types of comparing fractions worksheets available, their benefits, and how they can be used to enhance learning.
Introduction
Fraction worksheets are an invaluable resource for teachers and parents alike, providing a structured and engaging way to introduce, reinforce, and assess students’ understanding of fractions. Among the many types of fraction worksheets, those focusing on comparing fractions hold a special significance. Comparing fractions is a crucial skill that lays the foundation for understanding more complex mathematical concepts like adding, subtracting, multiplying, and dividing fractions.
Comparing fractions worksheets are designed to help students develop the ability to determine which fraction is greater, smaller, or equal to another fraction. This involves understanding the relationship between the numerator and denominator of a fraction, and how these values influence the overall size of the fraction. These worksheets can be tailored to different grade levels and learning abilities, providing a gradual progression from simple comparisons to more challenging scenarios.
The use of visual aids and real-world examples is crucial in making fraction comparison more intuitive and relatable for students. Fraction worksheets often incorporate diagrams, number lines, and real-life scenarios to help students visualize the concept and make connections between abstract mathematical concepts and their practical applications. These worksheets can be used for individual practice, small group activities, or as part of a larger lesson plan.
Types of Fraction Worksheets
Comparing fractions worksheets come in a variety of formats, each catering to different learning styles and skill levels. These worksheets can be broadly categorized based on the types of fractions they focus on, the methods employed for comparison, and the complexity of the problems. Some common types include⁚
- Comparing Fractions with Same Numerators⁚ These worksheets focus on comparing fractions with the same numerator but different denominators. Students are encouraged to visualize the fractions as parts of a whole and compare the sizes of the pieces.
- Comparing Fractions with Different Denominators⁚ These worksheets challenge students to compare fractions with different numerators and denominators. Students might need to find common denominators, use visual representations, or apply other strategies to determine which fraction is greater.
- Using Benchmark Fractions for Comparison⁚ These worksheets introduce students to benchmark fractions like 1/2, 1/4, and 3/4. Students are asked to compare fractions to these benchmarks to quickly determine their relative size.
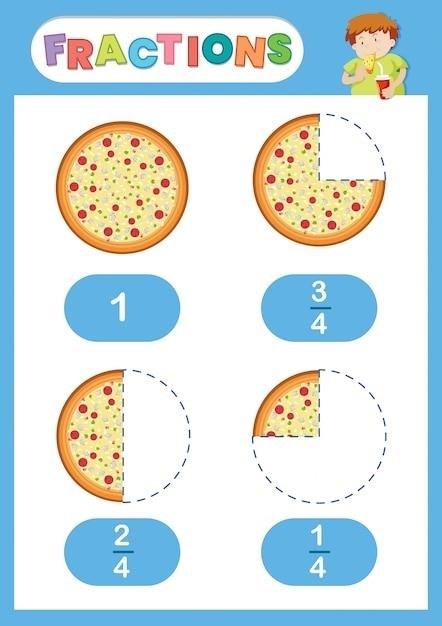
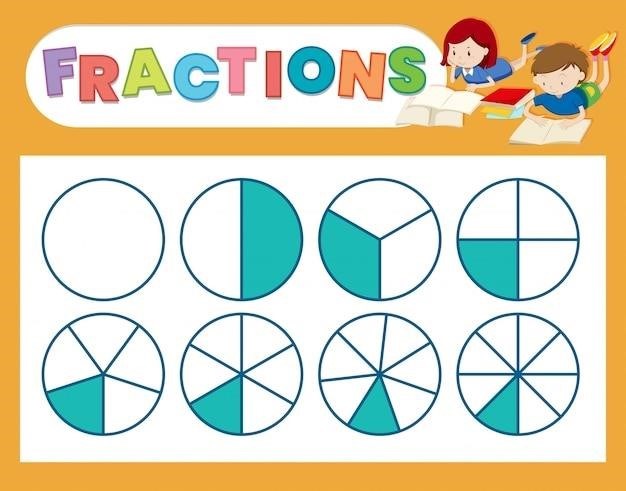
- Visual Representations for Comparing Fractions⁚ These worksheets utilize diagrams, number lines, and other visual aids to help students understand the concept of fraction comparison. Students might be asked to shade in parts of shapes or place fractions on a number line to compare their positions.
Fraction worksheets can also be differentiated based on the difficulty level, incorporating concepts like mixed numbers, improper fractions, and even decimal representations of fractions. By offering a diverse range of worksheet types, educators can provide students with a comprehensive and engaging learning experience.
Comparing Fractions with Same Numerators
Comparing fractions with the same numerator presents a relatively straightforward concept, making it an ideal starting point for introducing fraction comparison. Worksheets designed for this purpose typically feature a series of fractions with identical numerators but differing denominators. Students are tasked with identifying which fraction represents a larger portion of a whole.
The key to understanding this type of comparison lies in recognizing that when the numerators are the same, the fraction with the smaller denominator represents a larger portion. For instance, 1/2 is greater than 1/4 because the denominator 2 indicates that the whole is divided into fewer, larger parts. Visual aids like fraction bars or number lines can be helpful in illustrating this concept.
Comparing fractions with the same numerator provides a foundational understanding of the relationship between the denominator and the size of a fraction. It sets the stage for more complex comparisons involving fractions with different numerators and denominators, ultimately strengthening students’ grasp of fraction concepts.
Comparing Fractions with Different Denominators
Comparing fractions with different denominators poses a greater challenge than comparing fractions with the same numerator. This type of comparison requires students to understand the concept of equivalent fractions and the need for a common denominator. Worksheets designed for this purpose typically present pairs of fractions with different denominators, requiring students to determine which fraction is greater, less than, or equal to the other.
One common approach to comparing fractions with different denominators is finding a common denominator. This involves converting the fractions to equivalent fractions with the same denominator. For example, to compare 1/3 and 2/5, one would find the least common multiple of 3 and 5, which is 15. Then, 1/3 would be converted to 5/15 and 2/5 would be converted to 6/15. Now, with the same denominator, it is clear that 6/15 is greater than 5/15.
Another approach involves visualizing the fractions using models like fraction bars or number lines. This allows students to visually compare the relative sizes of the fractions. These worksheets provide valuable practice for students in developing a strong understanding of equivalent fractions and applying this knowledge to compare fractions with different denominators.
Using Benchmark Fractions for Comparison
Benchmark fractions, such as 1/2, 1/4, and 3/4, serve as helpful reference points for comparing fractions. By understanding the relative positions of these benchmark fractions on a number line, students can quickly estimate the value of other fractions. This strategy simplifies the process of comparing fractions, especially when dealing with unfamiliar fractions.
Worksheets that incorporate benchmark fractions often present a fraction and ask students to compare it to a benchmark fraction. For example, students might be asked to compare 2/5 to 1/2. By recognizing that 2/5 is less than 1/2, they can confidently determine the relationship between the two fractions. This method encourages students to develop a sense of fraction magnitude and rely less on rote calculations.
Benchmark fractions are particularly useful for comparing fractions with different denominators. By comparing a given fraction to a benchmark fraction, students can estimate its value relative to other fractions, even those with different denominators. This strategy promotes mental math skills and helps students develop a deeper understanding of fraction relationships.
Visual Representations for Comparing Fractions
Visual representations play a crucial role in making fraction concepts accessible and engaging for students. Comparing fractions worksheets often utilize various visual aids to help students grasp the relationship between different fractions. These visuals provide a concrete understanding of fraction magnitudes, making comparison tasks more intuitive;
One common visual representation is the use of fraction circles or fraction bars. These diagrams depict a whole divided into equal parts, representing different fractions. By comparing the shaded portions of the circles or bars, students can visually determine which fraction is greater or smaller. This method is particularly helpful for students who are still developing their understanding of fraction concepts.
Another effective visual representation is the number line. Fractions are plotted on the number line, allowing students to compare their positions. Fractions located further to the right on the number line represent larger values. This approach reinforces the concept of fraction order and provides a spatial context for understanding fraction relationships.
Fraction Worksheets for Different Grade Levels
Comparing fractions worksheets are designed to cater to students at various grade levels, ensuring appropriate challenges and learning experiences. The complexity of the worksheets increases as students progress through their academic journey. Early elementary grades focus on basic fraction concepts, using simple visuals and straightforward comparisons. As students advance to higher grades, the worksheets introduce more complex fractions, including mixed numbers, improper fractions, and comparisons involving unlike denominators.
For instance, worksheets for second graders might involve comparing fractions with the same denominator, often illustrated with pictures of divided shapes. Third-grade worksheets might introduce comparing fractions with different denominators, requiring students to find common denominators before making comparisons. Fourth and fifth-grade worksheets might delve into comparing mixed numbers, ordering fractions, and even introducing the concept of equivalent fractions.
The availability of worksheets tailored to specific grade levels allows teachers to select appropriate materials that align with the curriculum and cater to the diverse learning needs of their students. This ensures that students are presented with challenges that are both engaging and manageable, promoting a positive learning experience and fostering confidence in their fraction skills.
Real-World Applications of Fraction Comparison
While comparing fractions may seem like an abstract mathematical concept, it holds significant relevance in real-world scenarios. Understanding fraction comparison is crucial for everyday tasks and decision-making. For example, when baking, comparing fractions is essential for determining the correct proportions of ingredients. A recipe might call for 1/2 cup of flour and 1/4 cup of sugar, and understanding the relative sizes of these fractions is vital for a successful outcome.
Fraction comparison also plays a role in measuring and sharing resources. Imagine splitting a pizza equally among friends. To ensure fairness, it’s necessary to compare the sizes of the slices, ensuring that everyone receives an equal share. Similarly, in construction, comparing fractions is essential for calculating precise measurements and ensuring that materials are cut to the correct dimensions.
Beyond everyday scenarios, fraction comparison is vital in various fields, including finance, engineering, and science. Financial analysts use fractions to compare investment returns, while engineers rely on fractions to calculate precise measurements and tolerances. Scientists use fractions to analyze data and express the results of experiments. These examples highlight the practical relevance of fraction comparison and its significance in navigating the complexities of the real world.
Tips for Using Comparing Fractions Worksheets
Maximizing the effectiveness of comparing fractions worksheets requires thoughtful implementation. To ensure students grasp the concept and develop a strong foundation in fraction comparison, consider these tips⁚
Start with Visual Representations⁚ Introduce fraction comparison using visual aids like fraction circles, number lines, or fraction bars. These visual representations help students visualize the relative sizes of fractions and make the concept more tangible.
Gradual Progression⁚ Begin with simple comparing fractions worksheets that focus on fractions with the same denominator. As students gain confidence, gradually introduce fractions with different denominators and mixed numbers.
Real-World Connections⁚ Connect fraction comparison to real-world scenarios, such as sharing a pizza, measuring ingredients, or dividing a piece of cake. These real-life applications make the concept more relatable and meaningful for students.
Encourage Discussion and Collaboration⁚ Facilitate class discussions where students can share their strategies for comparing fractions. Encourage them to explain their reasoning and justify their answers.
Provide Feedback and Support⁚ Review students’ work on the worksheets, providing constructive feedback and addressing any misconceptions. Offer extra support to students who are struggling, ensuring that they have the opportunity to practice and solidify their understanding.
By following these tips, educators can effectively use comparing fractions worksheets to foster a deeper understanding of this important mathematical concept.
Comparing fractions is a fundamental skill in mathematics that lays the groundwork for more advanced mathematical concepts. Comparing fractions worksheets serve as invaluable tools for educators, providing a structured and engaging way to introduce, reinforce, and assess student understanding of this concept.
From simple visual representations to more complex problems involving mixed numbers and unlike denominators, these worksheets cater to various learning levels and offer flexibility in tailoring instruction. The use of real-world applications and the encouragement of discussion and collaboration ensure a deeper understanding and a more meaningful learning experience.
As students progress through these worksheets, they develop a strong foundation in comparing fractions, which empowers them to confidently tackle more complex mathematical challenges in the future. With the right approach and resources, comparing fractions worksheets can significantly contribute to students’ mathematical growth and success.